Absolute value is a versatile concept with practical applications in various real-life scenarios, such as determining the distance between two points, calculating the magnitude of velocity, measuring margin of error, reporting bank account balances, fitting puzzle pieces, estimating hiking elevation change, tracking stock price movements, and gauging the intensity of earthquakes.
Examples of Absolute Value
Here are 7 examples of absolute value:
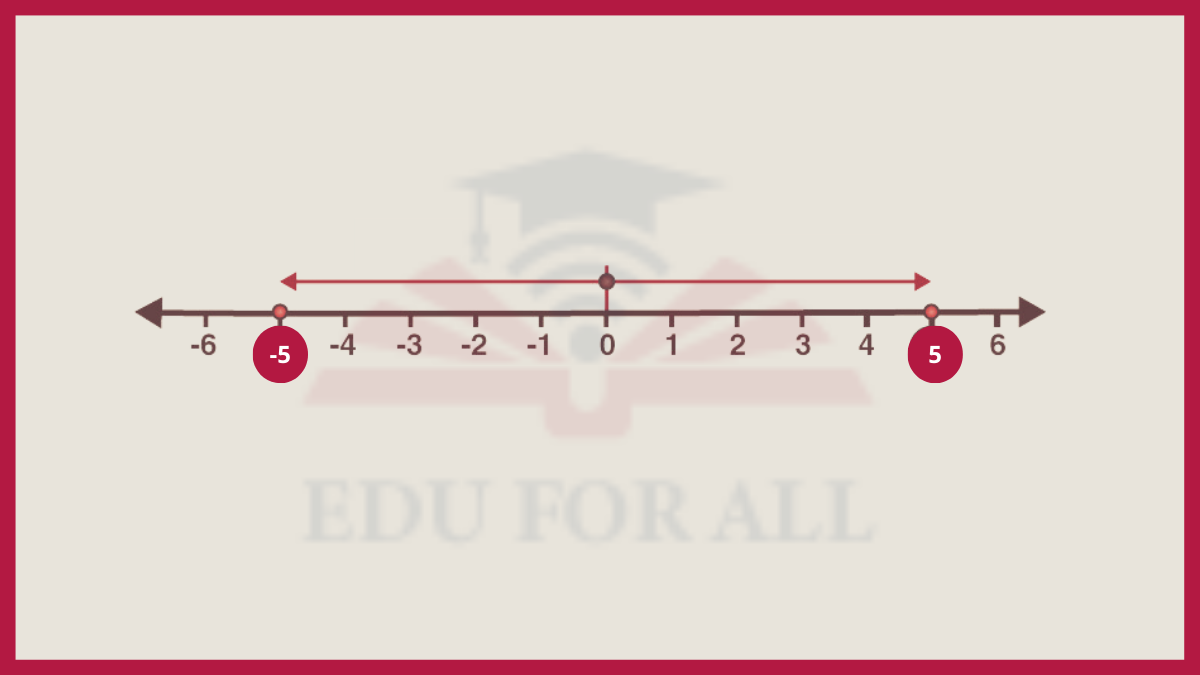
1. Distance Between Two Points
The absolute value of the difference between two numbers on a number line represents the distance between those points, regardless of direction. For example, the positions 3 and 5 are 2 units apart. |5 – 3| = |2| = 2, which calculates the magnitude of the distance. Without absolute value, just 5 – 3, direction would be included, wrongly implying one outranks the other.
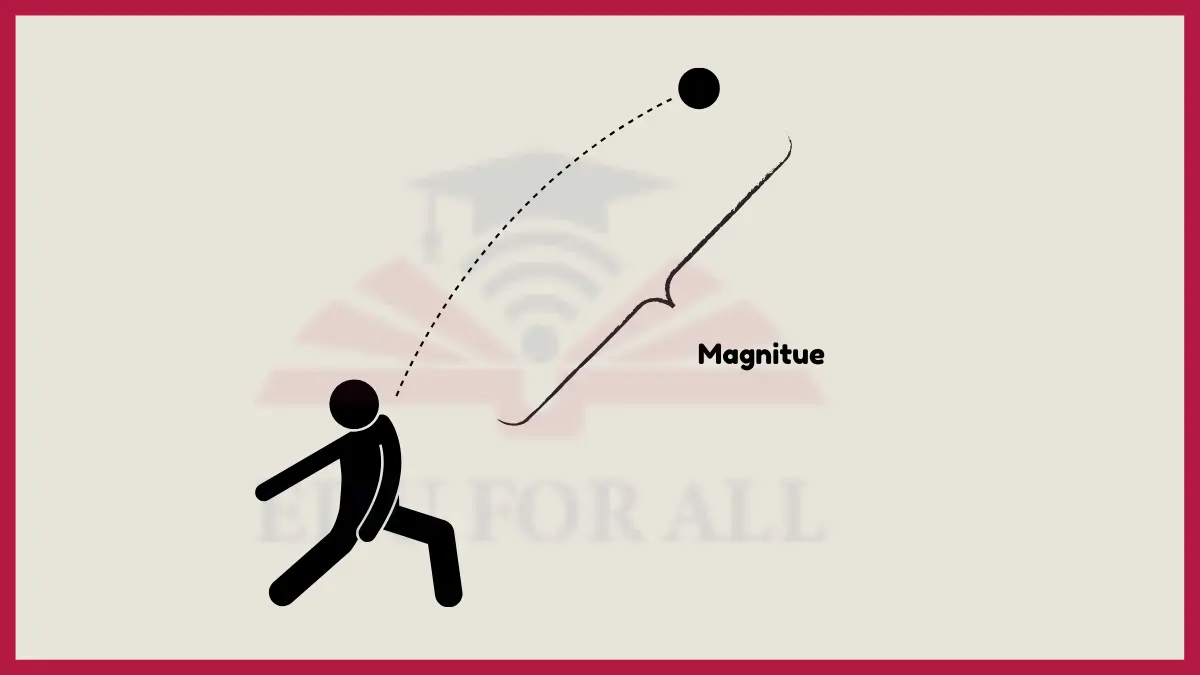
2. Magnitude of Velocity
In physics, velocity indicates both speed and direction of motion. However, with absolute value, |-25 meters/second| = |25 meters/second|. This gives only speed, by removing the negative sign that shows an opposite direction. Absolute value of velocity is useful for calculations involving kinetic energy, momentum, speed limits, race times, etc – things involving only magnitude, not direction.
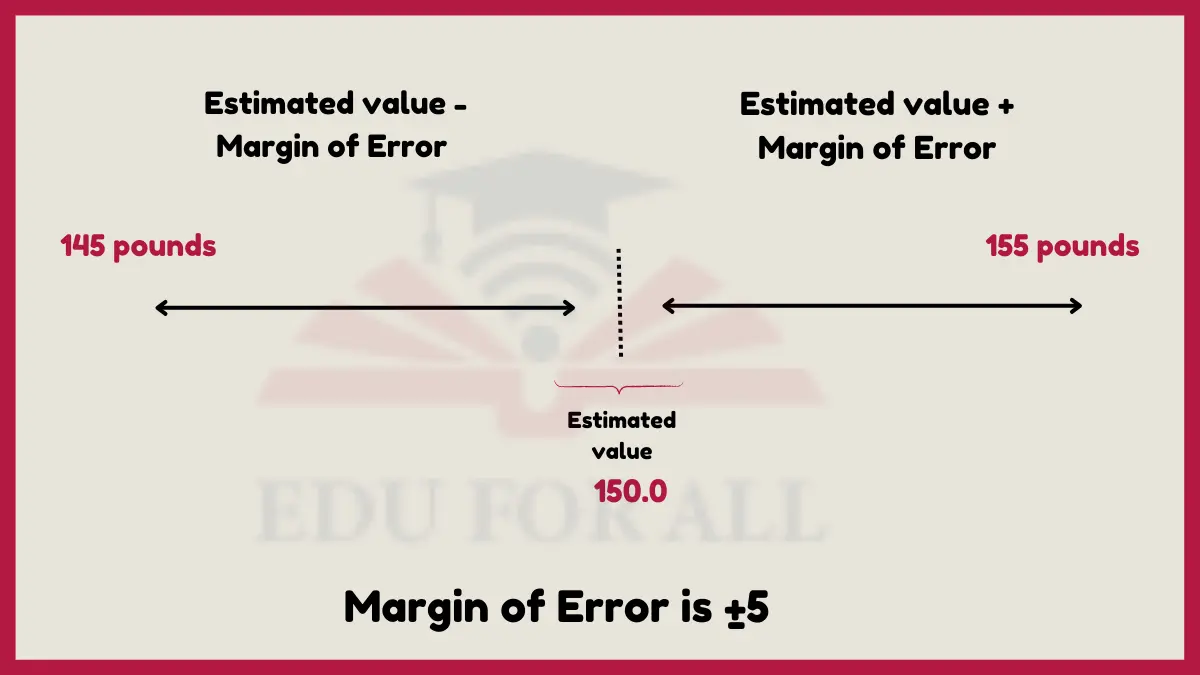
3. Margin of Error
When taking measurements, estimates may deviate from true values. The absolute difference between an estimate and actual number is called the margin of error. For example, if a building’s height is 50m and it gets estimated as 53m, error is |estimate – actual| = |53 – 50| = |3| = 3m. In statistics, smaller margins improve accuracy.
4. Bank Balance Reporting
When stating bank account balances, the absolute value amount gets reported, ignoring negatives. So if your account statement shows -$250, implying debt, its reported balance is |-$250| = |$250| = $250. This conveys amount owed in a positive form, without directionality.
5. Puzzle Piece Fitting
When fitting together puzzle pieces, orientation often flips, but shape/size stays consistent. A piece measuring |4 inches x 2 inches| will always measure |4 inches x 2 inches| after flipping, because absolute value fixes its dimensions regardless of right-side up vs upside down.
6. Hiking Elevation Change
When hiking, total ascent involves adding up all elevation gains (using absolute values), regardless of intermediate descents. If you descend 300ft then climb up 200ft, your total ascent is |-300 ft| + |200 ft| = 300 ft + 200 ft = 500 ft (using absolute values). This gives total change in elevation.
7. Stock Price Movement
In stocks, absolute value conveys price shift size regardless of up/down direction. If a stock drops from $25 to $20, it moved |$20 – $25| = |-$5| = $5. Without absolute value, it would imply $20 “greater” than $25, wrongly suggesting upward direction.
Examples of Absolute Value of A Number In Math
Here are a few Examples of absolute value of a number in math:
Positive numbers
- ∣3∣=3∣3∣=3: The absolute value of 3 is 3.
- ∣12∣=12∣12∣=12: The absolute value of 12 is 12.
- ∣9∣=9∣9∣=9: The absolute value of 9 is 9.
- ∣1.75∣=1.75∣1.75∣=1.75: The absolute value of 1.75 is 1.75.
- ∣7.2∣=7.2∣7.2∣=7.2: The absolute value of 7.2 is 7.2.
Negative numbers
- ∣−8∣=8∣−8∣=8: The absolute value of -8 is 8.
- ∣−2.5∣=2.5∣−2.5∣=2.5: The absolute value of -2.5 is 2.5.
- ∣−15∣=15∣−15∣=15: The absolute value of -15 is 15.
- ∣−100∣=100∣−100∣=100: The absolute value of -100 is 100.
Zero
∣0∣=0∣0∣=0: The absolute value of 0 is 0, since the distance from zero to itself is zero, regardless of direction.
Decimal numbers
- ∣−0.5∣=0.5∣−0.5∣=0.5: The absolute value of -0.5 is 0.5.
- ∣1.25∣=1.25∣1.25∣=1.25: The absolute value of 1.25 is 1.25.
- Expressions: 13. ∣−3+7∣=4∣−3+7∣=4: The absolute value of -3 + 7 is 4.
- ∣−10×2∣=20∣−10×2∣=20: The absolute value of -10 times 2 is 20.
- ∣−63∣=2∣∣3−6∣∣=2: The absolute value of −633−6 is 2.
Also Read

